Properties of angular momentum operators

Problem:

The angular momentum operators {J_x, J_y, J_z} are central to quantum theory.  States are classified according to the eigenvalues of these operators when J is conserved by the respective Hamiltonian H.
(a)  What condition(s) is (are) necessary for all eigenstates of H to be eigenstates of J? 
An eigenstate of J is usually specified by |j,m_z>,
where J²|j,m_z>  =  j(j + 1)ħ²|j,m_z> and J_z|j,m_z> = m_zħ|j,m_z>.
(b)  We can substitute J_x or J_y for J_z in (a).  However a state cannot be simultaneously an eigenstate of J_z and J_x.  Derive the commutation relation for the angular momentum operators J_x and J_z, (i.e.  [J_x,J_z] = -iħJ_y) from the definition of the linear momentum operator.
(c)  Prove that it is indeed possible for a state to be simultaneously an eigenstate of J² = J_x² + J_y² +J_z² and J_z. 

Problem:

Show that if any operator commutes with two of the components of an angular momentum operator, it commutes with the third.

Problem:

Consider the three spin matrices

(a)  Calculate the commutator of S_x and S_y.
(b)  What are the possible values we can get if we measure the spin along the x-axis?
(c)  Suppose we obtain the largest value when we measure the spin along the x-axis.  If we now measure the spin along the z-axis, what are the probabilities for the various outcomes?

 Solution:

• Concepts:
  The eigenvectors and eigenvalues of the angular momentum operator, spin 1
• Reasoning:
  We are asked to predict the outcomes of measurements on a spin 1 particle.
• Details of the calculation:
  (a)  The commutator is
  
  (b)  We can measure one of the eigenvalues of S_x, namely ħ, 0, or -ħ.
  (c)  The largest possible eigenvalue is ħ.
  To find the corresponding eigenvector expanded in terms of the eigenvectors of S_z we solve the system of equations
  .
  We obtain a = b/√2 = c.
  The normalized eigenvector is |1>_x = ½|1> + (1/√2)|0> + ½|-1>.
  The probabilities for measuring ħ and -ħ are ¼, and the probability of measuring 0 is ½.

Problem:

Consider the vector space E(k,j) spanned by vectors with the same k and j but different indices m.
(a)  What is the dimension of this space?
(b)  The space is globally invariant under the action of all components of J.  Any component of J acting on a vector in E(k,j) yields another vector in E(k,j).  What are the matrices of J_z, J₊, and J_- in E(k,j)?
(c)  Write down the matrices of J², J_z, J_x, and J_y for j = 0, ½, and 1.

Solution:

• Concepts:
  The eigenvectors and eigenvalues of the angular momentum operator
• Reasoning:
  The the vector space E(k,j) spanned by vectors with the same k and j is a subspace of the state space E(k,j,m) spanned by the eigenvectors of a complete set of commuting observables, where two of those observables are J² and J_z.  We can consider E(k,j,m) to be the direct sum of all orthogonal subspaces E(k,j) spanned by vectors with the same k and j but different indices m. 
• Details of the calculation:
  (a)  The dimension of the space is 2j + 1.  J² and J_z have common eigenvector.  Each eigenvalue of J² characterized by j is 2j + 1 fold degenerate, there are 2j + 1 possible values of m.
  (b)  J_z|k,j,m> = mħ|k,j,m>.
  J₊|k,j,m> = [j(j+1) - m(m+1)]^½ħ|k,j,m+1>.
  J_-|k,j,m> = [j(j+1) - m(m-1)]^½ħ|k,j,m-1>.
  The matrix elements in E(k,j) therefore are
  <k,j,m|J_z|k,j,m'> = mħδ_{m m'},
  <k,j,m|J_±|k,j,m'> = [j(j+1) - m'(m'±1)]^½ħ δ_{m m'±1}.
  The matrix elements are independent of the value of k, they depend only on j and m, they are "universal".  They do not depend on a particular physical system.
  (c)  j = 0:
  The space E(k,j) is one-dimensional, the only possible value for m is zero.  All matrices reduce to one element, which is zero.
  
  j = ½:
  In the { |+½>, |-½> } basis we have

  

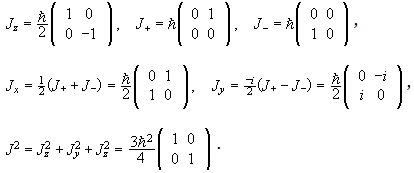
  j = 1:
  In the { |1>, |0>, |-1> } basis we have